

On July 10th 2024 I will host a one day event about topological solitons at Edge Hill University funded by an LMS celebrating new appointments grant. The day will consist of four talks about topological solitons and related topics. More details will be added once they have been confirmed.
The invited speakers are
If you would like to attend please fill out the form at https://forms.office.com/e/uT2pQV9Dqw, registration for the event is free. If there are any questions the please send me an email at calum.ross[at]edgehill[dot]ac[dot]uk, there is, very, limited funding available to cover some transport costs for speakers and any PhD students who wish to attend. Please get in touch if you would like ask about this support.
The full program will be confirmed nearer the time a rough outline is included below. All the talks will be held in the Tech Hub lecture theatre THG08. There will be a lunch provided at 13:00 in the Tech Hub foyer, and after the talks anyone who is staying in Ormskirk for the evening can go for dinner
| Time | Speaker | Topic/Title | Abstract/ Summary of the talk |
|---|---|---|---|
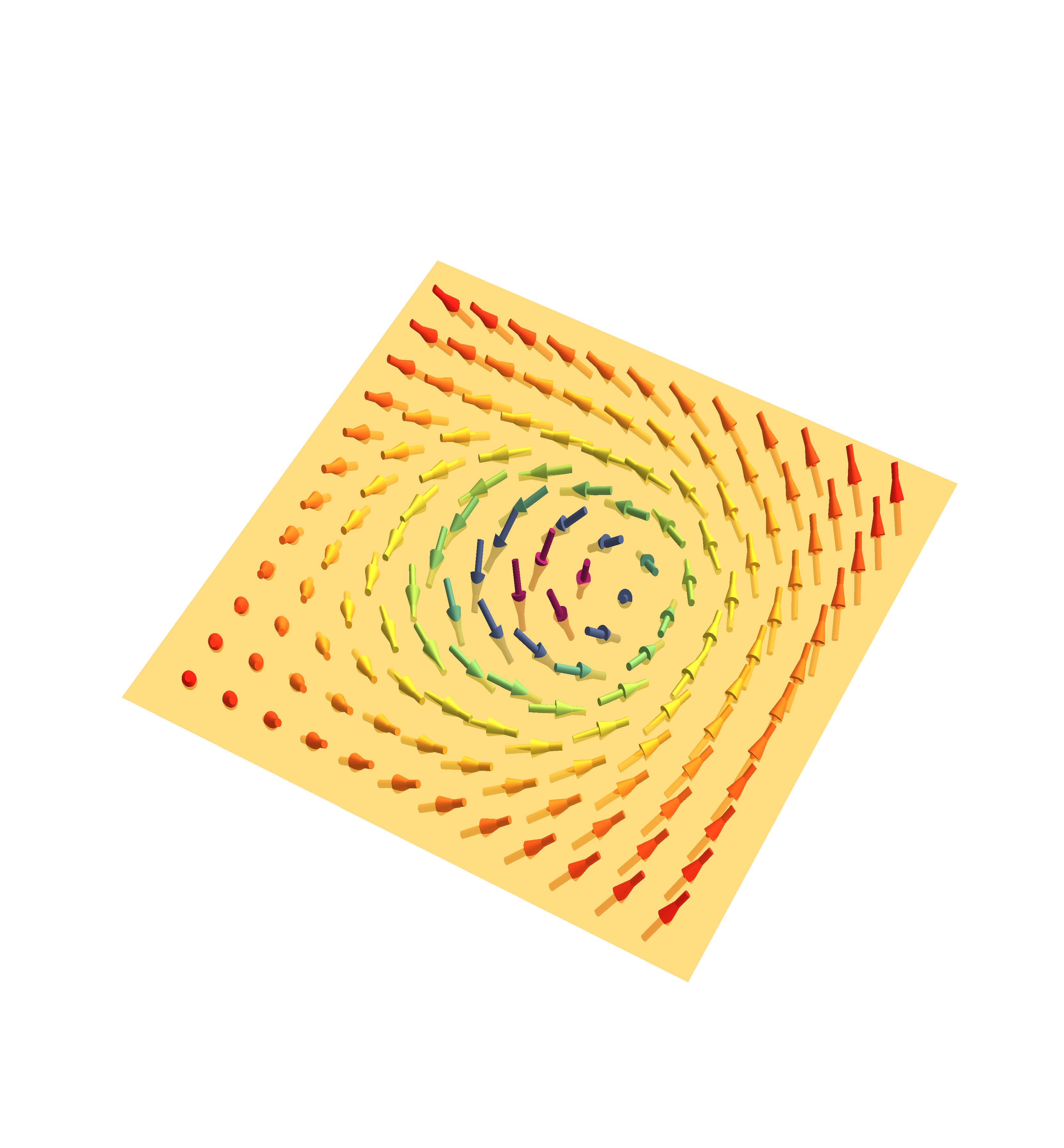
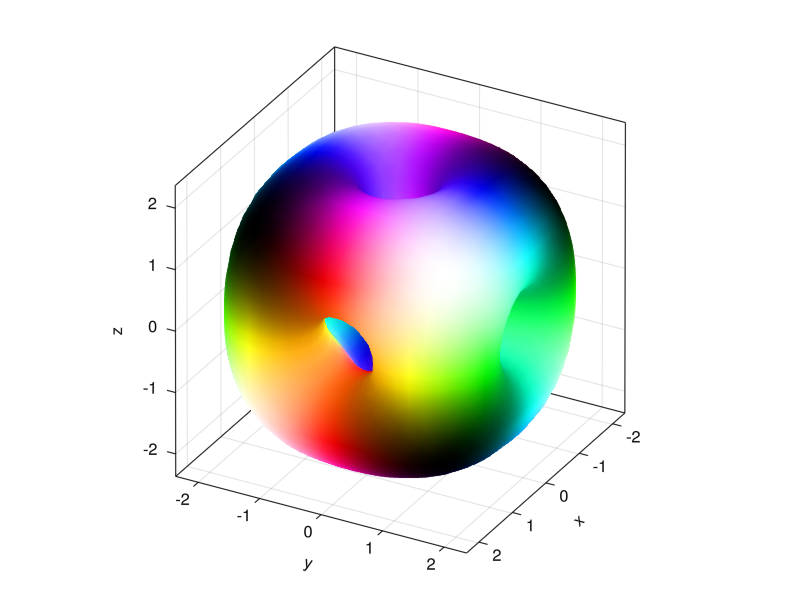
| 12:00 | Calum Ross (EHU) | Domain Wall Skyrmions | Chiral magnets are examples of materials which admit topologically non-trivial configurations, such as domain walls, spirals, and skyrmions. These have all been extensively studied, with domain walls and skyrmions both thought to have applications as information carriers. However, their response to external currents complicates this. A superposition of a domain wall and a skyrmion, called a domain wall skyrmion, may resolve this as the skyrmion is restricte to move along the domain wall. I will sketch out a mathematical model describing chiral magnets, demonstrate how we construct domain wall skyrmion configurations, and show some examples. This is based on joint work with Muneto Nitta and Yuki Amari. A recording of this talk is available on Youtube. |
| 13:00 | Lunch | ||
| 14:00 | Tom Winyard (Edinburgh) | Current driven dynamics of Magnetic Skyrmions | |
| 15:00 | Derek Harland (Leeds) | L2 geometry of hyperbolic monopoles | |
| 16:00 | Coffee Break | ||
| 16:30 | Guido Franchetti (Bath) | Harmonic Spinors on Kähler Manifolds | Harmonic spinors, that is solutions of the massless Dirac equation, have been the object of considerable interest from both the mathematical and physical communities. In the talk I will show how the rich structure of Kähler manifolds allows to recast the Dirac equation in a way which makes obtaining explicit solutions easier. The method will be applied to the Eguchi-Hanson manifold, for which we show how to reproduce known solutions, and to more general Ricci-flat Kähler manifolds obtained via the Calabi construction, for which we present new solutions. |
| 17:30 | Dinner? |
This meeting is supported by a celebrating new appointments grant from the London Mathematical Society (LMS).
